songbook
songbook.pdf ⟶
As we attain to the knowledge of simple things by way of compound things,
so must we reach to the knowledge of eternity by means of time,
which is nothing but the numbering of movement by “before” and “after.”
—Thomas Aquinas
Ornette Coleman's music allows all harmonies but provides unexpected tonal frames of reference. Similarly, these songs make use of an integrated tactus and form that encompass every possible tempo at any time-scale, but provide an unexpected gravitational frame for each rhythm.
The minimum, germinal composition is created by a response: a second event responds to a first. This pair can then receive a response of its own, and so forth. The composition of events is the organization of responses: the way in which responses are integrated into events of greater magnitude.
Our intuition expects the response to be equal to the call, and for the most part music meets our expectation. The most common song form in jazz is 32 bars long for this reason. A beat is played and answered by another beat, these beats are answered by two more to create one bar, this bar is answered by another, these bars are answered by two more, these four bars are answered by four more, these eight bars are answered by eight more, and finally, these sixteen bars are answered by sixteen more to complete the form.
While 32-bar song forms in 4/4 time are standard, other forms with local asymmetries are also common. For instance, the 12-bar blues form consists of an 8-bar call and a 4-bar response:
I hate to see that evening sun go down
I hate to see that evening sun go down
'cause my lovin' baby done left this town.
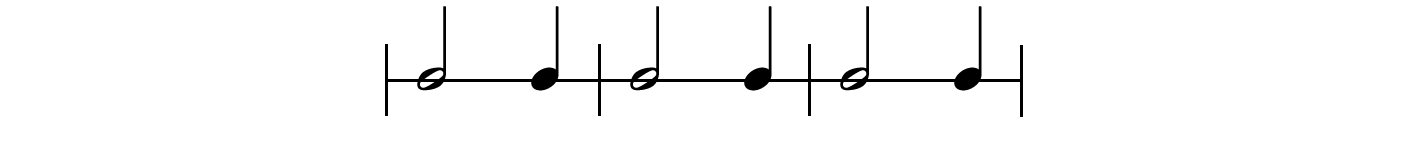
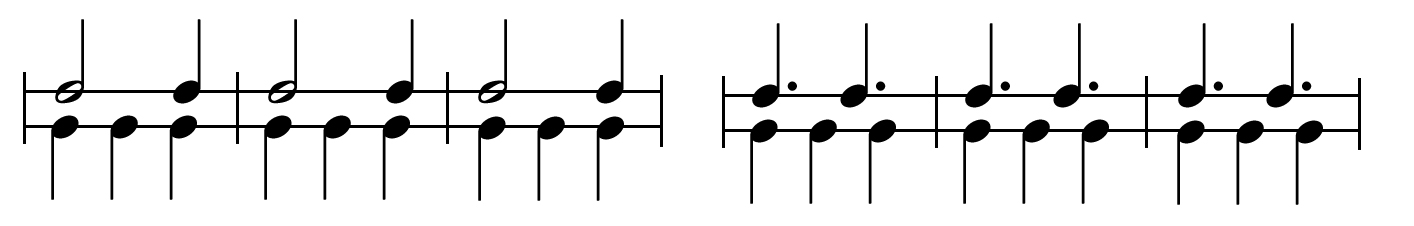
Tunes written by Jazz musicians often include comparable local asymmetries. On a smaller time scale, we see the same thing in any bar of 3/4 time: the second part of each bar, assuming that the first and third beats are the strong beats, is half the length of the first part:
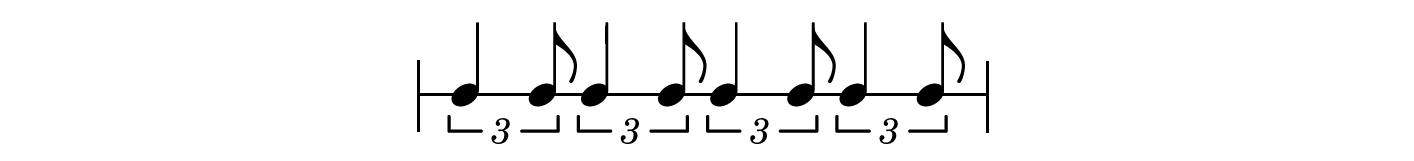
Going down to the time-scale of the beat, we again see the same thing in slow swing:
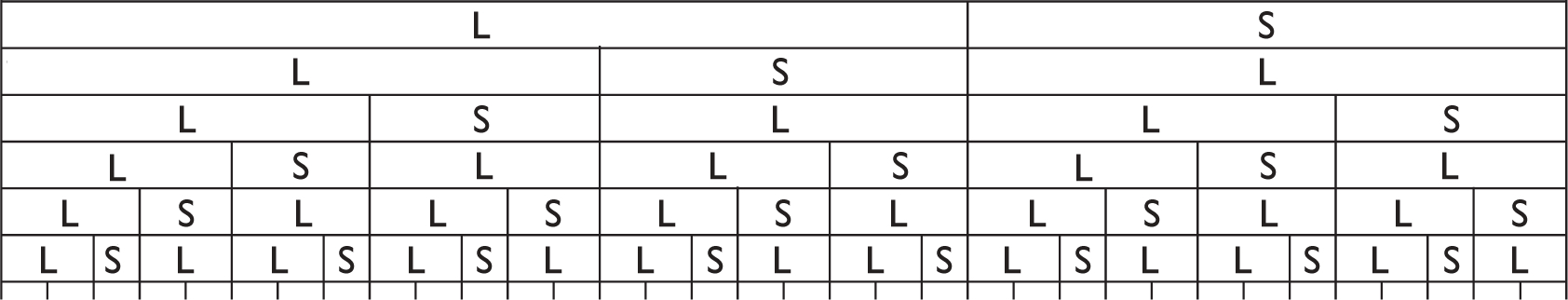
This songbook uses a second species of metric structure in which the response is shorter than the call, not merely at the level of the chorus, the barline, or the beat, but on every time-scale at once:
Or, the response is longer than the call on every time-scale at once:

In this species, every time-scale swings: just as upbeats in jazz lean into the next downbeat, propelling us into the future, phrases and sections of every scale of magnitude fall forward into the next.
The first species of meter exemplifies metric intuition. Even in the absence of a rhythm section the listener will provide a spontaneous symmetrical coordinate system in his mind: for any two events separated by an interval of time, a listener will measure further events off of an expected third event that happens after an equal interval of time. Traditional symmetrical song forms always yield the rhythmic interpretation that is expected/intended, because human intuition expects consequents to be of commensurate length to their antecedents.
Within the first species, an improviser always knows his location within the form and where he is going to land, without having to count. In a sense, the meter and the rhythm section remembers where the soloist is, on the soloist’s behalf. It provides a platform for a masterful display of variations, in the manner of the classical musics of the world. But jazz is more than a classical music because the best soloists surprise themselves. Between Armstrong and Ayler there was an evolution of musical language based on a rhythm section that manifests metric intuition, and a soloist that spills out beyond the confines of this intuition with impulsiveness, desire, hesitation, etc., to discover something unanticipated.
Ornette Coleman demonstrated that the figure/ground relationship of the soloist and rhythm section could provide risk just as effectively as any impulsiveness on the soloist’s part. In the absense of a song form, by the time a soloist gets to a note, the bassist has moved on, shifting the ground beneath the soloist's feet and providing an unforeseen 'question' to be answered in the next phrase. Unforeseen contingencies tie together points in time in a necessary way, making an event depend on an ensuing solution or making it accountable to a prior contingency. By contrast, time as a bridge to a solution is subjectively nullified in classical improvisation because there is no risk (assuming that one has achieved technical mastery).
By thinking of passing tones as the higher degrees of a chord, Charlie Parker was able to play a scale degree regardless of whether he was on the subdominant, dominant, or tonic of a given key. As this new degree of freedom was being clarified in modal improvisation, Ornette took it to its logical conclusion - regardless of what pitch a musician lands on at a given point in time, there will always be an interpretation. While this dovetailed with modernism's increasing understanding that meaning in music encompasses every possible structure, it did so as a lemma of a more fundamental point: that meaning occurs across the gamut of structure because it does not derive from structure, but from structure's ability to register contingency and the resolution of contingency. Structure is only the playground for contingency, and Ornette showed us the centrality of contingency by opening up this playground in the harmonic domain, and showing that the meaning was not found in the material itself, but the fact that the context and interpretation for it is never what one expects, particularly in the absence of a song form.
For a time it was possible to extrapolate from Ornette's superficially and conclude that risk is equal to one's elimination of composition. But total improvisation instead opened the door to an idiom completely uninhabited by contingency. When improvisation becomes total, a short circuit takes place. Unfettered intuition promptly reaches a homeostasis that can instantly absorb any event, no matter how abrupt. Song forms, while providing predictable harmony and phrasing, also served as a bulwark against this short-circuit. But they did so, not by providing harmony per se, but by providing a frame of reference provides the interpretation of the surprise.
This music takes a cue from Ornette in providing contingency by shifting the ground instead of the figure. But what Ornette Coleman's music did for harmony, it does for rhythm. Used systematically, asymmetrical meter reverses figure and ground: before the soloist can even begin to do something impulsive, the meter sideslips, on every time scale at once. The ground constantly shifts beneath the soloist’s feet, framing everything he plays in an unexpected light, giving it unexpected emphasis, forcing him to respond in a fresh way which will be similarly re-framed, etc. A third down never happens at the expected moment, so the soloist is always flying by the seat of his pants. All of his favorite devices have to be left at the door. If they are used, they will either fall flat, or, in the hands of a great soloist, slip into a completely unprecedented conclusion.
If we map the second species of meter onto the first, we see that the effect is either one of absolute postponement - as if the musicians never make it to the second barline:
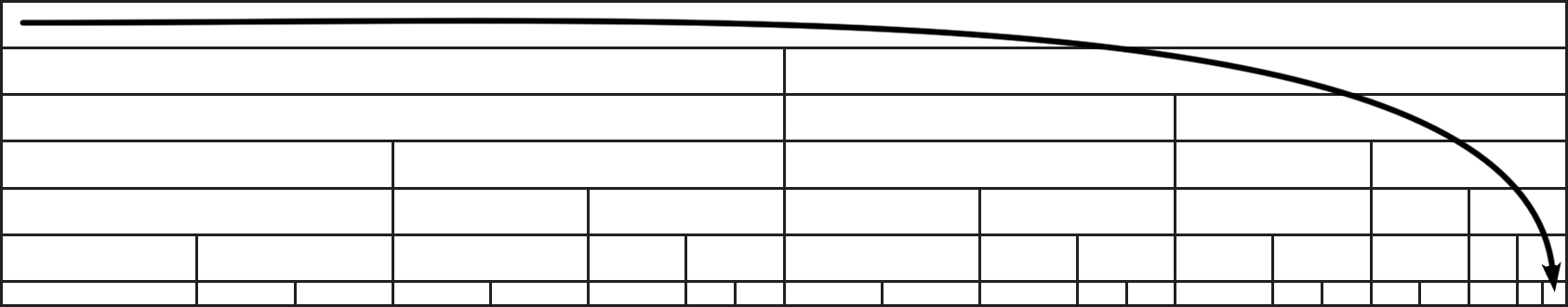
Or one of absolute anticipation - as if the musicians are perpetually accelerating past an exponentially proliferating barlines:
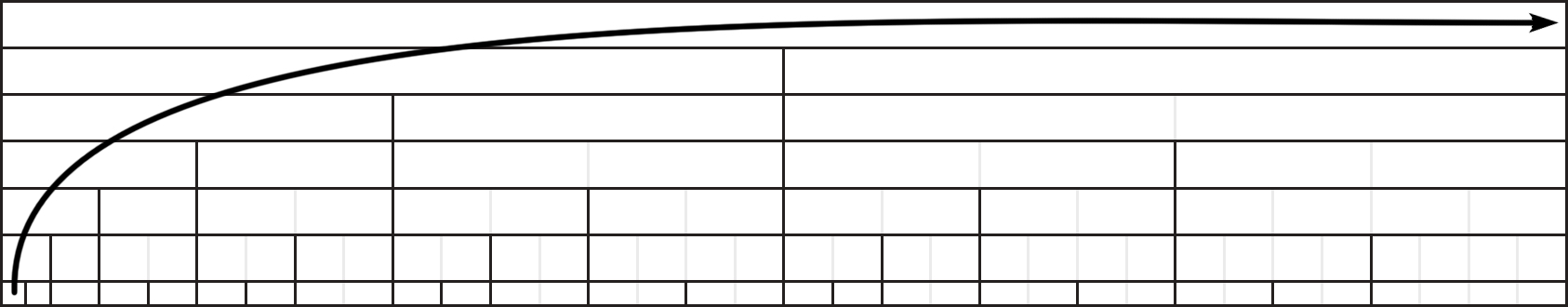
Repeating song forms create places in time that persist insofar as they return. If these places traditionally coalesce into something like a platform where the performer always knows where he is, antisymmetrical meter coalesces into something like a rabbit-hole in one case, and a gateless gate in the other. The soloist is immersed in a space where one never lands, but anything is possible. It yields a risk at every moment and magnitude. In a sense it is the topological counterpart of Armstrong’s gambit.
Half-time and double-time are built into symmetrical meter; it’s merely a matter of recognizing or prescinding from upbeats. Asymmetrical time-scales have further tempi as well; for instance, in 3/4 time, the two strong beats are elastic enough to be straightened out, revealing a compound of two tempi related by a 3:2 ratio:
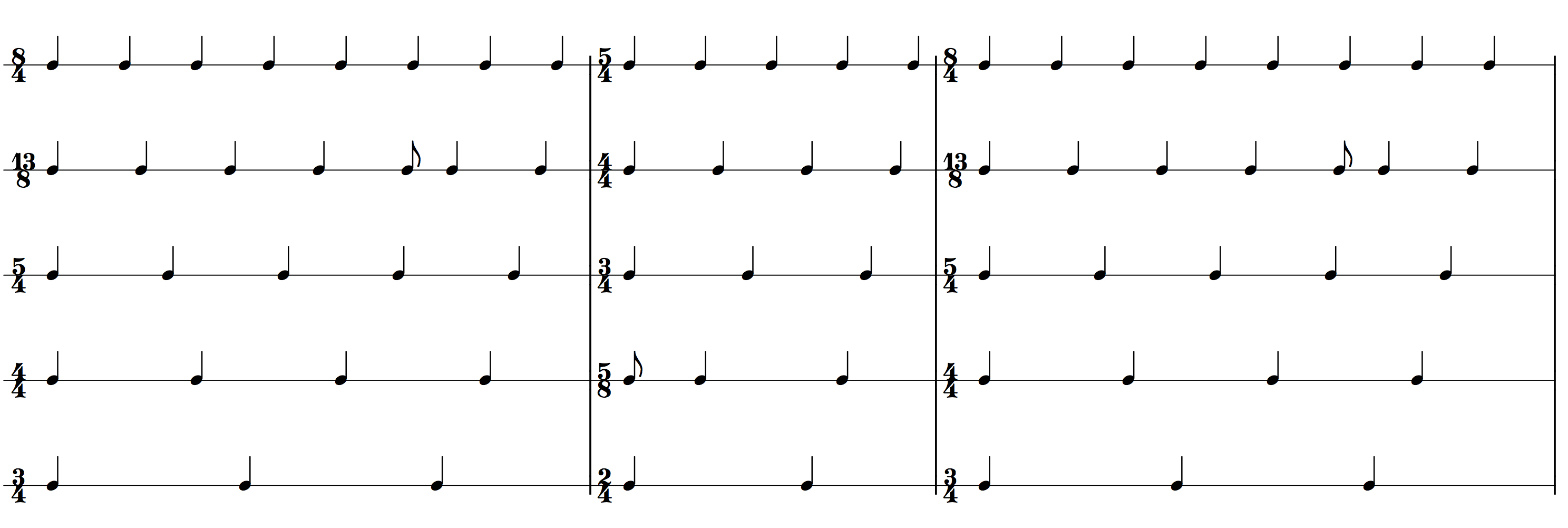
Analogously, the second species of metric structure is pervaded by a 3:4:5 ratio between time-scales:
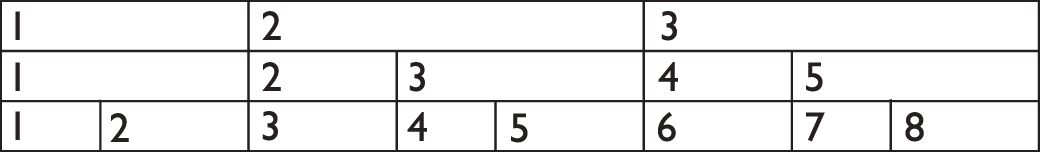

While one can play polyrhythms within symmetrical meter, in the second species they’re present in the grouping of pulses into slower pulses, just as double and half-time are always present in the first species. If Ornette’s music lifted the foreclosure on harmony represented by chord changes, this music lifts the foreclosure on tempi: no matter what speed you play at, it will have an interpretation, where interpretation here means gravitational coordinates, that is, a sense for whether each note is an upbeat or a downbeat relative to any other note, or a sense for whether a phrase is an antecedent or a consequent relative to any other phrase. The adjacent time scales are close enough in tempo that they act as an interpretive scaffolding for the entire continuum of pulse - that is, any pulse will be interpreted as one of the tempi inherent in the meter (perhaps slightly rushed or dragged). The result is that no matter what speed one plays at, one inhabits shared (and anisotropic) gravitational coordinates with everyone else. When combined with their double and half times, these inherent tempi produce a complete harmonic system of rhythm. The difference between the first species of meter and the second species is like the difference between harmony based only on octaves and harmony based on fifths, that is to say, the difference between a lack of harmony and a complete harmonic system.
 ⟵
⟵